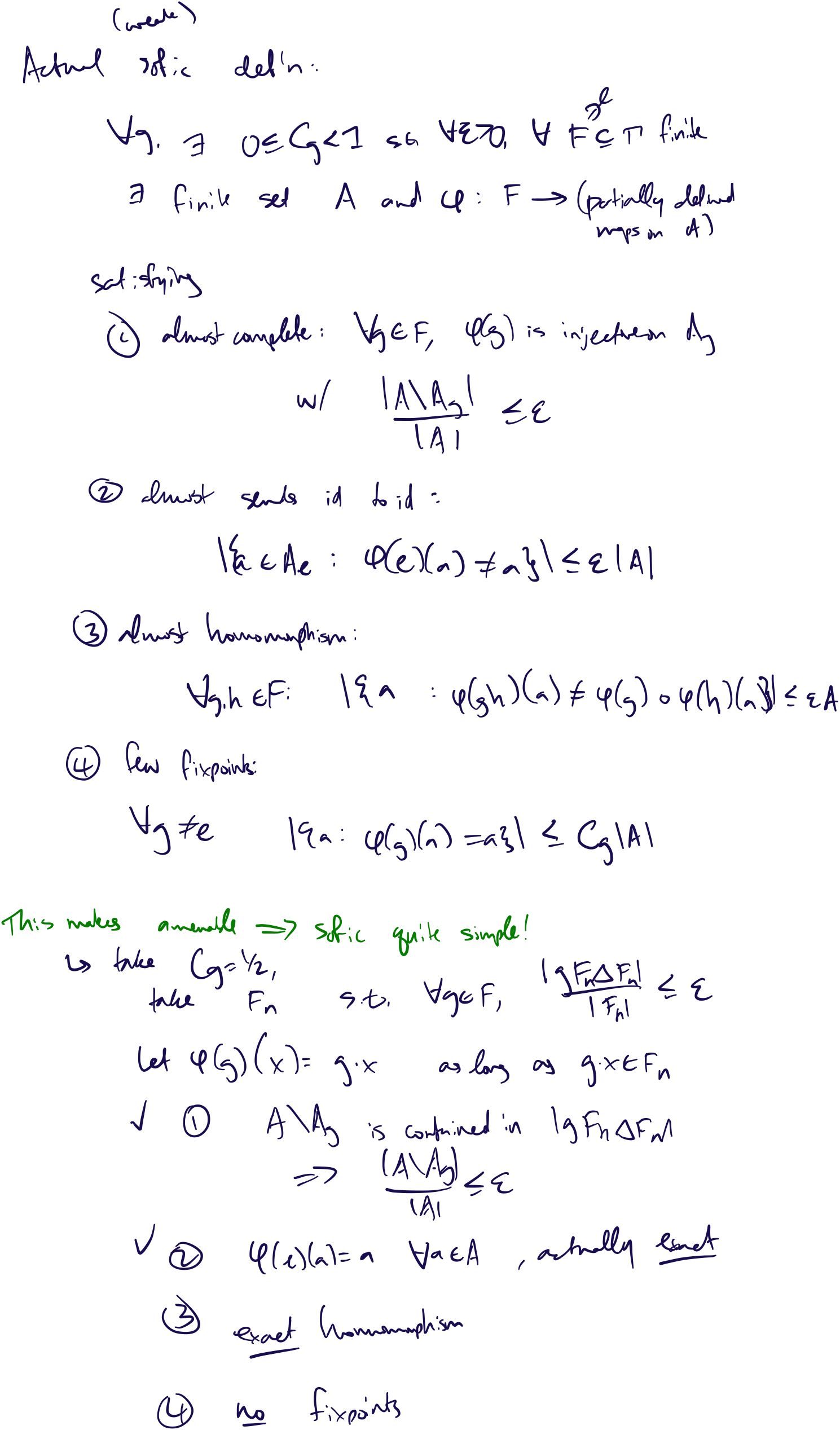amenable groups are sofic

#connection #fact
sofic group amenable group
(using definition of amenable groups via folner sequence)

Intuition

• The point is that for any finite $F\subset \Gamma$, there's a set $F_n$ in your folner sequence that's essentially fixed by $F$
  • That is, $F$ acts on this set $F_n$ by left action.
  • This induces almost a homomorphism! With some fudging at the (small) border.
• So, sofic groups are relax amenability by allowing (approximate) homomorphisms on arbitrary sets (not just a subset)

Proof

(Using definition from https://web.ma.utexas.edu/users/juschenko/files/soficgroups.pdf section 2.2)